Lab 3: Center of Pressure on a Plane Submerged Surface

Lab 3: Center of Pressure on a Plane Submerged Surface

Objectives:

We are performing this ab to:

• Find out the center of pressure of a plane object. The surface can be totally or partially submerged in the liquid.
• Make the clear comparison between locations of center of pressure w.r.t centroid.

Introduction:

Center of pressure is defined as the point where we apply the hydrostatic force. The center of pressure also varies as the distance of the object varies from the liquid surface.

Fig 1: Center of Pressure

Theory:

The center of pressure apparatus is shown in the figure 1 given above. I the figure, a differential force is acting along a differential area given as:

The moment of dF about O in the figure above is:

Two cases are shown in the figure above. In case 1, the object is partially merged while in case 2 the object is fully merged in the liquid. We will discuss them one by one.

Case 1:

dF is differentiable from h/cosθ to R2:

Integrating the equation for moment between the same limits yield:

Case 2:

dF is differentiable from R1 to R2:

Integrating the equation for moment between the same limits yield:

The center of pressure in each case could be found by dividing the moment by the force.

Procedure:

Perform the following procedure step by step:

• First make sure that the zero line on back planes is in line with the zero degree line on tank. Also level the base plate.
• Start pouring the water into the tank at the desired angle θ, which can be acquired by removing the counter balance weight.
• Now hang the hanger of weight and add water to the quadrant tank. Keep adding water until desired angle is achieved.
• Observe and record the weight and distance of the water.
• Now add more weight, add more water until exact angle is achieved.
• Again record the weight and distance of the water h.
• Add water until we get the specified angle 0˚, 10˚, 20˚ and 30˚.
• Repeat the steps for all the angles.

Observation and Calculation:

Inner radius = R₁ = 10 cm

Outer radius = R₂ = 10 cm

Lever arm = R₃ =30 cm

Inside tank width = B =7.5 cm

Smallest graduation of measure of distance to free surface h = 3.8 cm

Case #	0˚			10˚			20˚			30˚
	Mass hanger (g)	Distance to free surface h (cm)	Cd (cm)	Mass hanger (g)	Distance to free surface h (cm)	Cd (cm)	Mass hanger (g)	Distance to free surface h (cm)	Cd (cm)	Mass hanger (g)	Distance to free surface h (cm)	Cd (cm)
1	50	15.1	4.9	108	14	6.1	170	14.4	4.6	268	13.8	4.1
	70	15	5.5	158	12.6	7.3	220	13	6.3	288	13	4.9
	120	13.2	7	208	11.6	8.6	270	11.8	7.5	338	11.8	6.3
	170	11.8	8.2	258	10.6	9.6	330	10.6	8.7	358	11.2	6.9
2	270	9.6	10	358	9.8	10	470	8	10	658	6.2	10
	320	8.6	10	408	8	10	520	7	10	698	5.4	10
	370	7.8	10	458	7	10	570	6	10	740	4.8	10
	470	5.8	10	508	5.8	10	620	5.2	10	790	3.8	10

Using the formulas given, the center of pressure is found to be:

Case #	0˚		10˚		20˚		30˚
	Distance to free surface h (cm)	CP	Distance to free surface h (cm)	CP	Distance to free surface h (cm)	CP	Distance to free surface h (cm)	CP
1	15.1	18.37	14	7.8	14.4	25.1	13.8	43.2
	15	18.33	12.6	8.33	13	23.9	13	41.4
	13.2	17.73	11.6	8.7	11.8	22.9	11.8	38.8
	11.8	17.27	10.6	9.1	10.6	21.9	11.2	37.5
2	9.6	16.54	9.8	15.31	8	13.19	6.2	14.7
	8.6	16.3	8	15.33	7	11.1	5.4	14.6
	7.8	16.2	7	15.35	6	43.1	4.8	14.5
	5.8	15.9	5.8	15.38	5.2	18.7	3.8	14.1

Plots: The plots are shown below:

Case 1:

For 0˚:

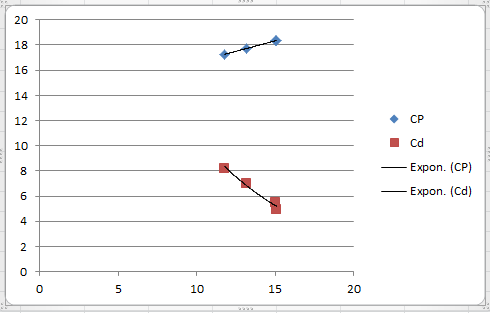
For 10˚:

For 20˚:

For 30˚:

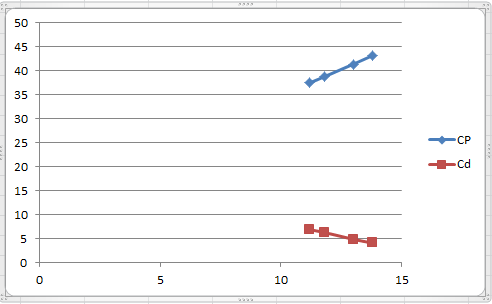
Case 2:

For 0˚:

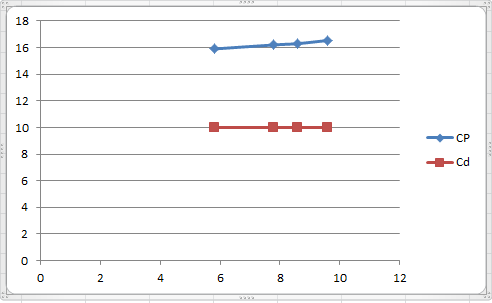
For 10˚:

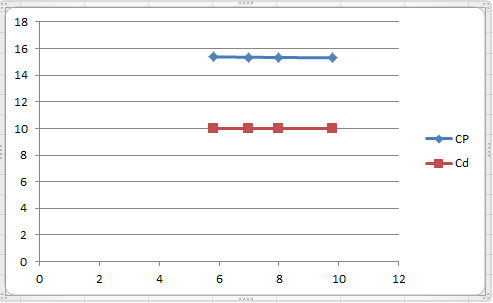
For 20˚:

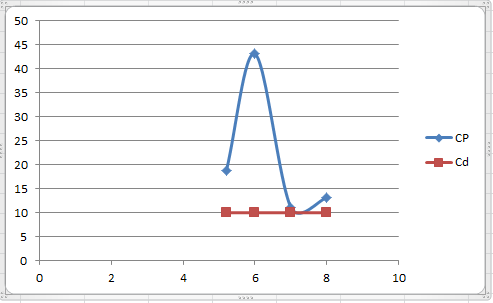
For 30˚:

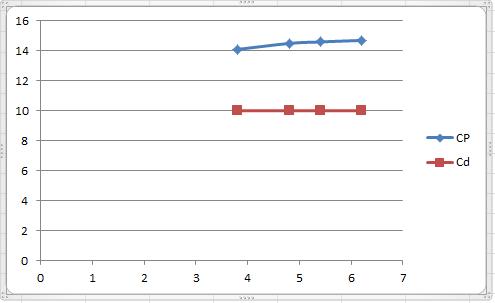
Results and Discussion:

The experiment was performed successfully and we determined that for each angle of inclination, the center of pressure falls above the centroid. The two coincide at 10˚ when the object is partially submerged while at 20˚ when the object is fully submerged.